- What is Bayes Theorem? Explain how Bayes Theorem can be utilized practically.(Solved)
What is Bayes Theorem? Explain how Bayes Theorem can be utilized practically.
Date posted: December 7, 2018. Answers (1)
- What is the addition rule of probability and for what type of events is it valid?(Solved)
What is the addition rule of probability and for what type of events is it valid?
Date posted: December 7, 2018. Answers (1)
- Under what conditions does P (A/B) = P (A)?(Solved)
Under what conditions does P (A/B) = P (A)?
Date posted: December 7, 2018. Answers (1)
- The purchasing department has analysed the number of orders placed by each of the 5 departments in the
company by type of this financial year as...(Solved)
The purchasing department has analysed the number of orders placed by each of the 5 departments in the
company by type of this financial year as given in the table below:
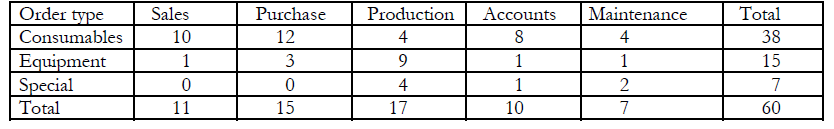
An error has been found in one of these orders.
Required:
i) Determine the probability that the incorrect order was not for consumables.
ii) Determine the probability that the incorrect order came from maintenance or production.
iii) Calculate the probability that the incorrect order was an equipment order from purchase.
Date posted: December 7, 2018. Answers (1)
- In a particular life insurance office, employees Simiyu, Juki, Waithera and Baraza have a diploma, with
Simiyu and Baraza also having a degree. Simiyu, Macharia, Waithera,...(Solved)
In a particular life insurance office, employees Simiyu, Juki, Waithera and Baraza have a diploma, with
Simiyu and Baraza also having a degree. Simiyu, Macharia, Waithera, Thuo, Mwanzia and Kungu are
associate members of the Chartered Insurance Institute (ACII) with Thuo and Mwanzia having a diploma.
Required:
Identifying set A as those employees with diploma, set C as those employees who are ACII and set D as
having a degree:
i) Specify the elements of sets A, C and D.
ii) Draw a Venn diagram representing sets A, C and D, together with their known elements.
iii) What special relationship exists between sets A and D?
iv) Specify the elements of the following sets and for each set, state in words what information is being
conveyed: A C; D C and D C .
v) What will be a suitable universal set for this situation?
Date posted: December 7, 2018. Answers (1)
- Explain briefly some of the advantages of the standard normal distribution(Solved)
Explain briefly some of the advantages of the standard normal distribution.
Date posted: December 7, 2018. Answers (1)
- Grear Tyre Company has just developed a new steel-belted radial tyre that will be sold through a chain of discount stores.
Because the tyre is a...(Solved)
Grear Tyre Company has just developed a new steel-belted radial tyre that will be sold through a chain of discount stores.
Because the tyre is a new product, the company‟s management believes that the mileage guarantee offered
with the tyre will be an important factor in the consumer acceptance of product. Before finalizing the tyre mileage guarantee policy, the actual road test with the tyres shows that the mean tyre
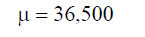
data collected indicate that a normal distribution is a reasonable assumption.
Required:
i) Grear Tyre Company will distribute the tyres if 20 per cent of the tyres manufactured can be expected
to last more than 40,000 kilometers. Should the company distribute the tyres?
ii) The company will provide a discount on a new set of tyres if the mileage on the original tyres does not exceed the mileage stated on the guarantee.
What should the guarantee mileage be if the company wants no more than 10% of the tyres to be
eligible for the discount?
Date posted: December 7, 2018. Answers (1)
- The past records of Salama Industries indicate that 4 out of 10 of the company?s orders are for
export. Further, their records indicate that 48 percent...(Solved)
The past records of Salama Industries indicate that 4 out of 10 of the company‟s orders are for
export. Further, their records indicate that 48 percent of all orders are for export in one particular
quarter. They expect to satisfy about 80 orders in the next financial quarter.
Required:
i) Determine the probability that they will break their previous export record
ii) Explain why you have used the approach you have chosen to solve part (i) above.
Date posted: December 7, 2018. Answers (1)
- An electric utility company has found out that the weekly number of occurrences of lightning striking the
transformers is a Poisson distribution with mean 0.4.
Required:
i) The...(Solved)
An electric utility company has found out that the weekly number of occurrences of lightning striking the
transformers is a Poisson distribution with mean 0.4.
Required:
i) The probability that no transformer will be struck in a week.
ii) The probability that at most two transformers will be struck in a week.
Date posted: December 7, 2018. Answers (1)
- In a certain factory that employs 500 men, 2% of all employees have a minor accident in a given year. Of
these, 30% had safety instructions...(Solved)
In a certain factory that employs 500 men, 2% of all employees have a minor accident in a given year. Of
these, 30% had safety instructions whereas 80% of all employees had no safety instructions.
Required:
Find the probability of an employee being accident-free given that he had:
i) no safety instructions.
ii) safety instructions
Date posted: December 7, 2018. Answers (1)
- State clearly what is meant by two events being statistically independent(Solved)
State clearly what is meant by two events being statistically independent.
Date posted: December 7, 2018. Answers (1)
- A firm has four plants scattered around the city producing the same homogeneous item at all plants. The first plant produces 30 per cent of...(Solved)
A firm has four plants scattered around the city producing the same homogeneous item at all plants. The first plant produces 30 per cent of the total production, second plant 25 per cent, third plant 35 per cent and the fourth plant 10 per cent. The firm has a single warehouse in the city for storing the finished product of all the plants without any distinction. From the past performance records on the proportion of defectives, it has been found that 5 per cent, 10 per cent, 15 per cent and 20 per cent from the items produced at plants 1, 2, 3, and 4 respectively are defective. Before the shipment of the items to a dealer, one unit is selected and found defective. What is the probability that the item was produced in plant 3?
Date posted: December 7, 2018. Answers (1)
- A firm recently submitted a bid for a turnkey project for a 500 MW power plant. If its main competitor submits a bid, the chances...(Solved)
A firm recently submitted a bid for a turnkey project for a 500 MW power plant. If its main competitor submits a bid, the chances of bid being awarded to the firm is 0.3. If the main competitor doesn't bid, there is a
3/4 chance of the firm getting the contract. There is a 0.50 chance that the main competitor will bid.
i) What is the probability of the firm‟s getting the contract?
ii) What is the probability that the competitor‟s bid given that the firm's bid is awarded?
Date posted: December 7, 2018. Answers (1)
- Computer analysis of satellite data has correctly forecast locations of economic oil deposits 80% of the time.
The last 24 oil wells drilled produced only 8...(Solved)
Computer analysis of satellite data has correctly forecast locations of economic oil deposits 80% of the time.
The last 24 oil wells drilled produced only 8 wells that were economic. The latest analysis indicates economic quantities at a particular location. What is the probability that the well will produce economic quantities of oil?
Date posted: December 7, 2018. Answers (1)
- The following table gives a bi-variate frequency distribution of 50 managers according to their age and salary
(in rupees).(Solved)
The following table gives a bi-variate frequency distribution of 50 managers according to their age and salary
(in rupees).
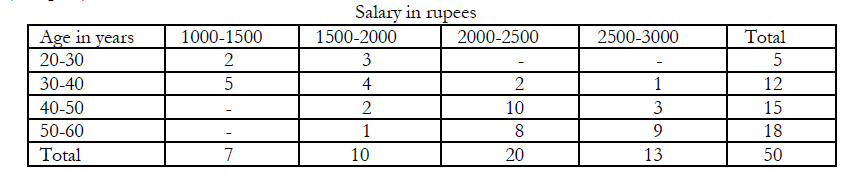
If a manager is chosen at random from the above distribution, find the chance that;
(i) he is in the age group of 30-40 and earns more than Rs.1500,
(ii) his earnings are in the range of Rs.2000-2500 and is less than 50 years old.
Date posted: December 7, 2018. Answers (1)
- Three groups of children contain respectively 3 girls and 1 boy; 2 girls and 2 boys; 1 girl and 3 boys. One child is selected...(Solved)
Three groups of children contain respectively 3 girls and 1 boy; 2 girls and 2 boys; 1 girl and 3 boys. One child is selected at random from each group, show that the chance that the three selected, consist of 1 girl and 2 boys is 13/32.
Date posted: December 7, 2018. Answers (1)
- A problem is given to three managers A, B, C whose chances of solving are ½, ?, ¼ respectively. What is the probability that the...(Solved)
A problem is given to three managers A, B, C whose chances of solving are ½, ⅓, ¼ respectively. What is the probability that the problem will be solved?
Date posted: December 7, 2018. Answers (1)
- Moving averages are often used in an effort to identify movements in share prices. Approximate monthly
closing prices (in Sh. per share) for Toys Children Ltd....(Solved)
Moving averages are often used in an effort to identify movements in share prices. Approximate monthly
closing prices (in Sh. per share) for Toys Children Ltd. for December 2000 through November 2001 are
shown below:

Date posted: December 6, 2018. Answers (1)
- The table below reports the annual net salary amounts for an accountant who began employment with an
auditing firm in 1995. In addition, the values of...(Solved)
The table below reports the annual net salary amounts for an accountant who began employment with an
auditing firm in 1995. In addition, the values of the Consumer Price Index (CPI) for 1995 through 1999 is
shown.

Required:
Determine whether there is a difference in percentage salary increase between 1995 and 1999 in terms of
stated (current) shillings amounts and in terms of constant (deflated salary, using CPI) shillings.
Date posted: December 6, 2018. Answers (1)
- Explain the following terms as used in index numbers:
i) Quantity indices.
ii) Base year.
iii) Chain index numbers.
iv) Retail price index.(Solved)
Explain the following terms as used in index numbers:
i) Quantity indices.
ii) Base year.
iii) Chain index numbers.
iv) Retail price index.
Date posted: December 6, 2018. Answers (1)