
When a body is projected vertically upwards, it decelerates uniformly due to gravity until its velocity reduces to zero at maximum height. After attaining the maximum height, the body then falls back with an increasing velocity. The body must be given an initial velocity and attains a final velocity of zero at its maximum height. Note that the sign of ‘g’ is negative for a vertical projection. This is because the body moves against gravity.
Hence the three equations of linear motion become:
v=u-gt, (from v=u+at)
h=ut-½gt2, (from s=ut+½gt2)
v2 =u2-2gh, (from v2 =u2 -2as)
But at maximum height hmax, v=0. Thus, the three equations reduce to:
i. gt=u,
ii. h=ut-½gt2
iii. u2 =2gh.
From equation (i), the time taken to attain the maximum height is given by;
t=u/g.
Similarly, the initial velocity u and the maximum height attained by the body hmax can be expressed as:
u=gt=(2ghmax)½
And hmax=ut-½gt2 =u2/2g.
When the body finally falls back to its point of projection, the displacement of the body will be zero. Substituting this in equation (ii), we obtain;
0=ut-½gt2
Therefore, 0=t(2u-gt)
And t=0 or t=2u/g, where t=0 is the time at the start of the projection and,
t is this is the total time of flight i.e for both upward projection and fall back. Note that the total time of flight is twice the time taken to attain maximum height.
also, the velocity of the body just before hitting its point of projection as it falls back is the same in magnitude but in opposite direction to its initial velocity; v=-u.
sharon kalunda answered the question on April 24, 2019 at 05:53
- Describe free fall.(Solved)
Describe free fall.
Date posted: April 24, 2019. Answers (1)
- Using a tick-timer tape show the patterns for the following motions;
a)A body moving at constant velocity.
b)A body moving with increasing velocity (accelerating).
c)A body moving with...(Solved)
Using a tick-timer tape show the patterns for the following motions;
a)A body moving at constant velocity.
b)A body moving with increasing velocity (accelerating).
c)A body moving with decreasing velocity (decelerating).
In each case show the direction of motion.
Date posted: April 24, 2019. Answers (1)
- Describe the following Velocity-time graphs:(Solved)
Describe the following Velocity-time graphs:
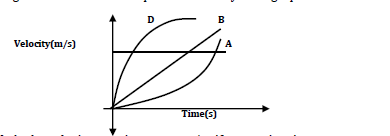
Date posted: April 24, 2019. Answers (1)
- Explain the dangers of static charges(Solved)
Explain the dangers of static charges
Date posted: April 23, 2019. Answers (1)
- In the figure below x=30cm, y=6cm, effort E=60N, A1=4cm2 and A2=12cm2.(Solved)
In the figure below x=30cm, y=6cm, effort E=60N, A1=4cm2 and A2=12cm2.

Calculate:
a) The force F exerted on the liquid at A1.
b) The velocity ratio of the system.
c) The maximum load that can be raised by the system.
Date posted: April 23, 2019. Answers (1)
- A box of mass 30kg is pushed up an inclined plane of length 14m using a force of 130N as shown below:(Solved)
A box of mass 30kg is pushed up an inclined plane of length 14m using a force of 130N as shown below:
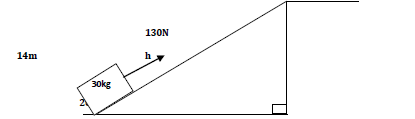
If the track is inclined at an angle of 200, calculate:
a) The height of the platform.
b) Workdone by the force of 130N.
Date posted: April 23, 2019. Answers (1)
- Draw a flow chart to show the energy transformation in a hydroelectric power station:(Solved)
Draw a flow chart to show the energy transformation in a hydroelectric power station:
Date posted: April 23, 2019. Answers (1)
- State the characteristics energy:(Solved)
State the characteristics energy:
Date posted: April 23, 2019. Answers (1)
- The mass of a density bottle is 20g when empty and 45g when full of water. When full of mercury, its mass is 360g. Calculate...(Solved)
The mass of a density bottle is 20g when empty and 45g when full of water. When full of mercury, its mass is 360g. Calculate the density of mercury.
Date posted: April 23, 2019. Answers (1)
- Derive the three equations of linear motion.(Solved)
Derive the three equations of linear motion.
Date posted: April 23, 2019. Answers (1)
- The figure below represents part of a tape pulled through a ticker-timer by a trolley moving down an inclined plane. If the frequency of the...(Solved)
The figure below represents part of a tape pulled through a ticker-timer by a trolley moving down an inclined plane. If the frequency of the ticker-timer is 50Hz, calculate the acceleration of the trolley.

Date posted: April 23, 2019. Answers (1)
- A paper tape was attached to a moving trolley and allowed to run through a ticker-timer. The figure below shows a section of the tape.(Solved)
A paper tape was attached to a moving trolley and allowed to run through a ticker-timer. The figure below shows a section of the tape.

If the frequency of the ticker-timer is 20Hz, determine:
a) The velocity between AB and CD.
b) The acceleration of the trolley.
Date posted: April 23, 2019. Answers (1)
- A body is made to change its velocity from 20m/s to 36m/s in 0.1s. What is the acceleration produced?(Solved)
A body is made to change its velocity from 20m/s to 36m/s in 0.1s. What is the acceleration produced?
Date posted: April 23, 2019. Answers (1)
- Outline the three common types of motion:(Solved)
Outline the three common types of motion:
Date posted: April 23, 2019. Answers (1)
- A machine with a velocity ratio of 30 moves a load of 300N when an effort of 200N is applied. Calculate the efficiency of the...(Solved)
A machine with a velocity ratio of 30 moves a load of 300N when an effort of 200N is applied. Calculate the efficiency of the machine.
Date posted: April 22, 2019. Answers (1)
- Explain why a man using a parachute falls through air slowly while a stone falls through air very fast(Solved)
Explain why a man using a parachute falls through air slowly while a stone falls through air very fast.
Date posted: April 22, 2019. Answers (1)
- A man makes a deeper marks while walking on a soft ground in a high heeled shoes than in a flat shoes. Explain(Solved)
A man makes a deeper marks while walking on a soft ground in a high heeled shoes than in a flat shoes. Explain
Date posted: April 22, 2019. Answers (1)
- Explain how temperature affects Brownian motion(Solved)
Explain how temperature affects Brownian motion.
Date posted: April 18, 2019. Answers (1)
- State the applications of extended light sources(Solved)
State the applications of extended light sources
Date posted: April 15, 2019. Answers (1)
- State the advantage of the Pinhole Camera over the Lens Camera(Solved)
State the advantage of the Pinhole Camera over the Lens Camera
Date posted: April 15, 2019. Answers (1)