- The figure below represent a square based right pyramid ABCDV. AB = 10cm,AV = BV = CV = DV = 15cm.(Solved)
The figure below represent a square based right pyramid ABCDV. AB = 10cm,AV = BV = CV = DV = 15cm.
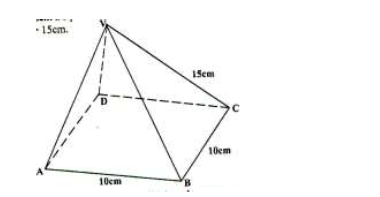
Calculate the angle between AV and the base ABCD to the decimal place.
Date posted: May 6, 2019. Answers (1)
- Solve for x without using mathematical tables or calculators.(Solved)
Solve for x without using mathematical tables or calculators.
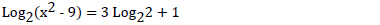
Date posted: May 6, 2019. Answers (1)
- Expand (4 - x)7 upto to the term in x4. Hence find the appropriate value of (3.8)7.(Solved)
Expand (4 - x)7 upto to the term in x4. Hence find the appropriate value of (3.8)7.
Date posted: May 6, 2019. Answers (1)
- In a transformation, an object with area 9cm2 is mapped onto an image whose area is 54cm2. Given that the matrix of transformation is (Solved)
In a transformation, an object with area 9cm2 is mapped onto an image whose area is 54cm2. Given that the matrix of transformation is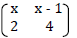 
find the value of x.
Date posted: May 6, 2019. Answers (1)
- Determine the amplitude and period of the function 3y = 6sin (2x - 30).(Solved)
Determine the amplitude and period of the function 3y = 6sin (2x - 30).
Date posted: May 6, 2019. Answers (1)
- The table shows the frequency distribution of marks scored by students in a test.(Solved)
The table shows the frequency distribution of marks scored by students in a test.
Determine the median mark correct to one decimal point.
Date posted: May 6, 2019. Answers (1)
- The quantities P, Q and R are such that P varies directly as Q and inversely as the square of R. Given that P =...(Solved)
The quantities P, Q and R are such that P varies directly as Q and inversely as the square of R. Given that P = 2 when Q = 12 and R = 6. Determine the equation connecting the three.
Date posted: May 6, 2019. Answers (1)
- In the figure below PQR is the diameter of the circle centre O. Angle QPR = 200 and angle QTR = 800(Solved)
In the figure below PQR is the diameter of the circle centre O. Angle QPR = 200 and angle QTR = 800
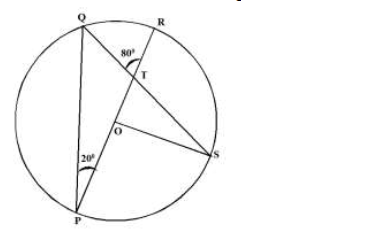
Determine the size of
a) Reflex angle POS
b) Angle OSQ
Date posted: May 6, 2019. Answers (1)
- The first term of an arithmetic sequence is 5 and the common difference is 2.(Solved)
The first term of an arithmetic sequence is 5 and the common difference is 2.
a) List the first six terms of the sequence.
b) Determine the sum of the first 40 terms of the sequence.
Date posted: May 6, 2019. Answers (1)
- The length of two similar iron bars A and B were given as 10.5m and 8.2m. Calculate the maximum possible difference
in length between the two...(Solved)
The length of two similar iron bars A and B were given as 10.5m and 8.2m. Calculate the maximum possible difference
in length between the two bars.
Date posted: May 6, 2019. Answers (1)
- The figure below shows a right pyramid VEFGHK. The base EFGHK is a regular pentagon. EO = 7cm and VE = 12cm.(Solved)
The figure below shows a right pyramid VEFGHK. The base EFGHK is a regular pentagon. EO = 7cm and VE = 12cm.
Calculate
a) The perimeter of the base to 2 decimal places.
b) The length VO to 2 decimal places.
c) The angle which edge VF makes with the edge FE.
d) The volume of the pyramid to 2 decimal places
Date posted: May 6, 2019. Answers (1)
- The figure below represents a game sanctuary in the shape of a quadrilateral in which AB = 30km,AE = 20km and CE = 45km (Solved)
The figure below represents a game sanctuary in the shape of a quadrilateral in which AB = 30km,AE = 20km and CE = 45km 0, 0 and 0.
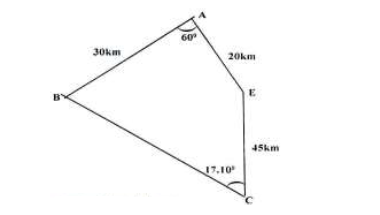
Calculate
a) The side BC correct to 2 decimal places.
b) The angle ABE to 1 decimal place.
c) The area of the game sanctuary in hectares correct to 2 decimal places.
Date posted: May 6, 2019. Answers (1)
- A calf runs in a straight line towards a cow with a velocity of vm/s after t seconds given by v = t (8 -...(Solved)
A calf runs in a straight line towards a cow with a velocity of vm/s after t seconds given by v = t (8 - t).
a) Complete the table below
b) Calculate the distance covered by the calf.
i) Using eight trapezia of equal width.
ii) Using the exact method.
Date posted: May 6, 2019. Answers (1)
- The percentage marks obtained by 40 students in a test are as under:
85, 30, 49, 62, 17, 84, 24, 15, 82, 61, 74, 38, 27,...(Solved)
The percentage marks obtained by 40 students in a test are as under:
85, 30, 49, 62, 17, 84, 24, 15, 82, 61, 74, 38, 27, 13, 44, 72, 61, 49, 38, 23,
90, 32, 67, 18, 45, 58, 22, 46, 37, 39, 43, 55, 62, 30, 46, 59, 41, 26, 34 and 47.
a) Prepare a grouped frequency table from the above data using a class width of 10.
b) Use 49.5 as the working mean and estimate the mean from the grouped frequency table.
c) Prepare a cumulative frequency table and draw the cumulative frequency curve on the grid of squares provided.
Date posted: May 6, 2019. Answers (1)
- Use this velocity - time graph which represents the motion of a car for 10 seconds, to find:(Solved)
Use this velocity - time graph which represents the motion of a car for 10 seconds, to find:
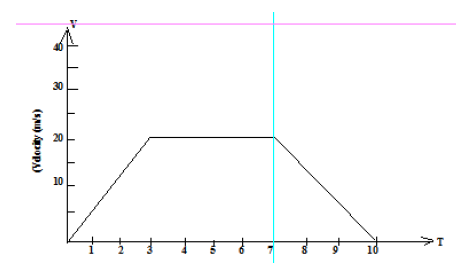
a) The rate of acceleration.
b) The rate of retardation.
c) The total distance travelled.
d) The total distance travelled during the first 4 seconds.
e) The average speed maintained during this journey.
f) The distance travelled at the constant speed.
Date posted: May 6, 2019. Answers (1)
- A line T passes through points (-3, -5) and (3, -6) and is perpendicular to a line l at (-2, -2).(Solved)
A line T passes through points (-3, -5) and (3, -6) and is perpendicular to a line l at (-2, -2).
a) Find the equation of l.
b) Find the equation of T in the form ax + by = c where a, b and c are constants.
c) Given that another line Q is parallel to T and passes through (1, -3) find x and y intercepts of Q.
d) Find the points of intersection of L and Q.
Date posted: May 6, 2019. Answers (1)
- Maina can do a piece of work in 12 hours. Muthui can do it in 20 hours. How long would it take Muthui to complete...(Solved)
Maina can do a piece of work in 12 hours. Muthui can do it in 20 hours. How long would it take Muthui to complete the work if Maina has been working for 7 hours.
Date posted: May 6, 2019. Answers (1)
- A particle moving in a straight line has its displacement x metres from the origin O at time t seconds defined by the
equation x =...(Solved)
A particle moving in a straight line has its displacement x metres from the origin O at time t seconds defined by the
equation x = t3 - 6t2 + 7. Determine the values of t for which the particle is momentarily at rest.
Date posted: May 6, 2019. Answers (1)
- A point A (-1, 3) is mapped onto A1(8, 12). Fidn the centre of enlargement given that the scale factor is 2.(Solved)
A point A (-1, 3) is mapped onto A1(8, 12). Fidn the centre of enlargement given that the scale factor is 2.
Date posted: May 6, 2019. Answers (1)
- A major arc of a circle substends an angle of 2500 at the centre of a circle. If the radius of the circle is 9.8cm...(Solved)
A major arc of a circle substends an angle of 2500 at the centre of a circle. If the radius of the circle is 9.8cm find the area of the minor sector. (Use 𝜋 = 22/7)
Date posted: May 6, 2019. Answers (1)