- a) MNQR is a rectangle in which MN = 5cm and NQ = 8cm. Construct the locus of a point P within the rectangle
which is...(Solved)
a) MNQR is a rectangle in which MN = 5cm and NQ = 8cm. Construct the locus of a point P within the rectangle
which is such that P is equidistant from sides NM and NQ
c) The locus of P in (a) above cuts MR at T. Draw a circle whose centre O is equidistant from the three sides of
triangle MNT and its radius is OM
c) In rectangle MNQR above, construct the locus of a variable point V such that 40o ≤ QVR ≤ 90o
Date posted: May 9, 2019. Answers (1)
- A triangle PQR whose vertices are P(2, 2), Q(5, 3) and R(4, 1) is mapped onto triangle P1Q1R1 by a transformation
whose matrix is(Solved)
A triangle PQR whose vertices are P(2, 2), Q(5, 3) and R(4, 1) is mapped onto triangle P1Q1R1 by a transformation
whose matrix is

a) On the grid provided below, draw triangle PQR and triangle P1Q1R1
b) Triangle P1Q1R1 is mapped onto a triangle whose vertices are P11(-2, -2), Q11(-5, -3) and R11(-4, -1)
i) Draw triangle P11Q11R11 on the same grid.
ii) Find the matrix representing transformation that maps triangle P1Q1R1 onto triangle P11Q11R11
c) Describe the transformation that maps PQR onto triangle P11Q11R11
Date posted: May 9, 2019. Answers (1)
- In 2001 the salaries of Gitonga and Cherop were sh.252000 per annum and sh.216000 per annum respectively. Their
employers decided to increase their salaries as follows.
Gitonga’s...(Solved)
In 2001 the salaries of Gitonga and Cherop were sh.252000 per annum and sh.216000 per annum respectively. Their
employers decided to increase their salaries as follows.
Gitonga’s employer decided to give him fixed annual increments throughout his employment period, with first
increment in January 2002.Cherop’s employer decided to give her increments of 8% compounded annually throughout her employment period with the first increment in January 2002.
a) If Gitonga annual salary in 2009 was sh.346080, calculate his annual increment.
b) How much money in total did Gitonga earn from is salaries from 1st January 2001 to 31st December 2009 ?
c) Determine Cherop’s monthly salary of August 2009.
d) How much money in total did Cherop earn from her salaries from 1st January 2001 to 31st December 2009.
e) Determine the difference between Gitonga’s and Cherop’s average yearly earnings from 1st January 2001 to 31st
December 2009.
Date posted: May 9, 2019. Answers (1)
- In the figure below, AB is a diameter of the circle. Chord PQ intersects AB at N. BR which is a tangent to the circle...(Solved)
In the figure below, AB is a diameter of the circle. Chord PQ intersects AB at N. BR which is a tangent to the circle at B
meets PQ produced at R.
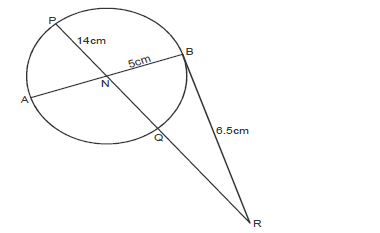
Given that PN = 14cm, NB = 5cm and BR = 6.5cm.
Calculate :
a) NR
b) AN
Date posted: May 9, 2019. Answers (1)
- Given OX = 4i + j + 3k and OY = 7i - 5j + k. If M is the mid-point of line XY, determine...(Solved)
Given OX = 4i + j + 3k and OY = 7i - 5j + k. If M is the mid-point of line XY, determine the modulus of XM.
Date posted: May 9, 2019. Answers (1)
- The table below is part of the tax table for monthly income for the year 2007.(Solved)
The table below is part of the tax table for monthly income for the year 2007.
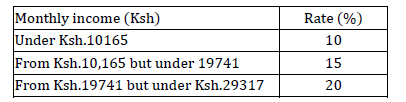
In that year, Adan’s monthly gross tax was Ksh.2,885. Calculate his monthly income.
Date posted: May 9, 2019. Answers (1)
- An aeroplane took off from an airport at (68oN, 86oE) and flew due West for a distance of 1000 nautical miles before landing. Find to...(Solved)
An aeroplane took off from an airport at (68oN, 86oE) and flew due West for a distance of 1000 nautical miles before landing. Find to the nearest degree, the coordinates of the place where the plane landed.
Date posted: May 9, 2019. Answers (1)
- Two students are selected at random from a class of 15 boys and 10 girls. Find the probability that(Solved)
Two students are selected at random from a class of 15 boys and 10 girls. Find the probability that
a) they are both boys
b) one is a boy and the other is a girl
Date posted: May 9, 2019. Answers (1)
- Simplify leaving your answer in surd form(Solved)
Simplify leaving your answer in surd form
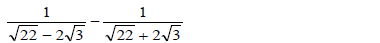
Date posted: May 9, 2019. Answers (1)
- a) Complete the following table for the function y = 6 + 3x - 2x2(Solved)
a) Complete the following table for the function y = 6 + 3x - 2x2
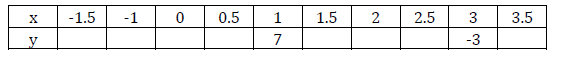
b) Using the completed table and the trapezoidal rule with 10 strips, estimate the area bounded by the curve and the
lines y = -8 and x = -1.5
Date posted: May 9, 2019. Answers (1)
- The coordinates of the ends of a diameter of a circle are (6, 4) and (-2, 2). If the centre of the circle is Q,...(Solved)
The coordinates of the ends of a diameter of a circle are (6, 4) and (-2, 2). If the centre of the circle is Q, determine:
a) the coordinates of centre Q
b) the equation of the circle expressing it in the form x2 + y2 + ax + by + c = 0 where a, b and c are constants.
Date posted: May 9, 2019. Answers (1)
- The length and width of a rectangle measured to the nearest centimetre are 10cm and 6cm respectively. Calculate the
percentage error in the area giving your...(Solved)
The length and width of a rectangle measured to the nearest centimetre are 10cm and 6cm respectively. Calculate the
percentage error in the area giving your answer to 1 decimal place.
Date posted: May 9, 2019. Answers (1)
- Solve for x,given that;(Solved)
Solve for x,given that;

Date posted: May 9, 2019. Answers (1)
- Grade A tea is mixed with grade B tea. The cost per kg of grade A is Ksh.60 and that of grade B is Ksh.80....(Solved)
Grade A tea is mixed with grade B tea. The cost per kg of grade A is Ksh.60 and that of grade B is Ksh.80. Find the ratio
in which the two grades should be mixed in order to make a profit of 20% by selling 1kg of the mixture at Ksh.90.
Date posted: May 9, 2019. Answers (1)
- a) Determine the inverse of the matrix(Solved)
a) Determine the inverse of the matrix

b) Hence find the coordinates of the point of intersection of the lines x + 2y = 7 and -y + x = 1
Date posted: May 9, 2019. Answers (1)
- R is partly constant and partly varies as the square of q. When R = 5, q = 1 and R = 21 when q...(Solved)
R is partly constant and partly varies as the square of q. When R = 5, q = 1 and R = 21 when q = 3. Find the value of R
when q = 5
Date posted: May 9, 2019. Answers (1)
- Use logarithms to evaluate(Solved)
Use logarithms to evaluate

Date posted: May 9, 2019. Answers (1)
- PQRS is a trapezium where PQ is parallel to SR. PR and SQ intersect at X, so that SX = kSQ and PX = hPR...(Solved)
PQRS is a trapezium where PQ is parallel to SR. PR and SQ intersect at X, so that SX = kSQ and PX = hPR where k and h
are constants.
Vectors PQ = 3q and PS = s, SR = q
a) Show this information on a diagram.
b) Express vector SQ in terms of s and q
c) Express SX in terms of k, q and s
d) Express SX in terms of h, q and s
e) Obtain h and k
f) In what ratio does X divide SQ ?
Date posted: May 9, 2019. Answers (1)
- A particle starts from rest and moves in a straight line. Its velocity Vm/s is given by V = t2 - 3t + 2, where...(Solved)
A particle starts from rest and moves in a straight line. Its velocity Vm/s is given by V = t2 - 3t + 2, where t is the time in seconds moved from a point O.
Find :
a) i)the velocity when t = 3.
ii) the displacement from O when t = 3.
iii) the acceleration of the particle when t = 3.
b) At what time is the particle momentarily at rest ?
Date posted: May 9, 2019. Answers (1)
- In the figure below, ABC is a tangent to the circle, centre O. DOG is a diameter and angle DGF = 60o, angle DBC =...(Solved)
In the figure below, ABC is a tangent to the circle, centre O. DOG is a diameter and angle DGF = 60o, angle DBC = 48o and angle DFE = 25o. Giving reasons, find the size of angles :

) FED
ii) Obtuse FOB
iii) EBD
iv) BCD
v) OBE
Date posted: May 9, 2019. Answers (1)