- Given the matrix below is a singular matrix, find the values of x(Solved)
The matrix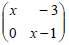 
is a singular matrix, find the values of x
Date posted: September 12, 2019. Answers (1)
- A field is 10m longer than its width. The area is 7200m2. What is the width?(Solved)
A field is 10m longer than its width. The area is 7200m2. What is the width?
Date posted: September 12, 2019. Answers (1)
- Find the expansion in ascending powers of x (Solved)
Find the expansion in ascending powers of x of 
up to the term in x2. Hence evaluate (0.99)7 to four significant figures.
Date posted: September 12, 2019. Answers (1)
- Given that Tan 750= 2+ v3 , hence determine Tan 150 leaving your answer in the form
a+ bvc(Solved)
Given that Tan 750= 2+ √3 , hence determine Tan 150 leaving your answer in the form
a+ b√c
Date posted: September 12, 2019. Answers (1)
- Make Q the subject of the formula(Solved)
Make Q the subject of the formula
Date posted: September 12, 2019. Answers (1)
- A point P divides AB in the ratio 7: -5 where A (2,-3, 4) and B (-4, 7,-2). Find the coordinates of P.(Solved)
A point P divides AB in the ratio 7: -5 where A (2,-3, 4) and B (-4, 7,-2). Find the coordinates of P.
Date posted: September 12, 2019. Answers (1)
- PQR is an equilateral triangle of sides 3cm. S is a variable point on the same side of PQ as R and on the same...(Solved)
PQR is an equilateral triangle of sides 3cm. S is a variable point on the same side of PQ as R and on the same plane such that angle PSQ=300. Locate by construction the possible location of S
(use a ruler and a pair of compasses only)
Date posted: September 12, 2019. Answers (1)
- Use logarithms to evaluate(Solved)
Use logarithms to evaluate
Date posted: September 12, 2019. Answers (1)
- Simplify completely.(Solved)
Simplify completely.
Date posted: September 12, 2019. Answers (1)
- A form four mathematics teacher originally worked out the mean mark of her fourty students to be fourty one.(Solved)
A form four mathematics teacher originally worked out the mean mark of her fourty students to be fourty one. After correction of the quiz. She added some marks to Timina, Chobola and Cheloti in the ration of 5:2:3 respectively. If the new mean marks for the students increased by 1.5 determine how many marks Cheloti was added than Chobola.
Date posted: September 12, 2019. Answers (1)
- The diagram below shows a straight line intersecting the curve y = (x – 1)2 + 4(Solved)
The diagram below shows a straight line intersecting the curve y = (x – 1)2 + 4 at the points P and Q. The line also cuts the x – axis at (7, 0) and y – axis at (0, 7)
(a) Find the equation of the straight line in the form y = mx + c.
(b) Find the co-ordinates of P and Q
(c) Calculate the area of the shaded region.
Date posted: September 11, 2019. Answers (1)
- Boeing 707 plane flies from Nairobi to South Africa. It has 600m2 of cabin space and can carry
5000kg of luggage.(Solved)
Boeing 707 plane flies from Nairobi to South Africa. It has 600m2 of cabin space and can carry
5000kg of luggage. An economy class passenger gets 3m2 of space is allowed 20kg of luggage.
A first class passenger gets 4m2 of space and is allowed 50kg of luggage. There must be space for At least 50 economy class passengers.
Let x be the number of economy seats and y be the number of first class seats
(i) List down all inequalities that satisfy these conditions.
(ii) Use the grid provided below to represent the region where x and y must lie.
(iii) The profit per flight from an economy seat is sh.4000 and from a first class seat is sh.10000
Use your graph to determine the allocation of seats which will give maximum profit.
Date posted: September 11, 2019. Answers (1)
- Three towns are located on the earth’s surface at the co-ordinates P(150N, 450E)(Solved)
Three towns are located on the earth’s surface at the co-ordinates P(150N, 450E), Q(300S, 450E) and R(150N, 900E). A plane A flies from P to Q in 6 hours 40 minutes using the shortest route between the two towns. Another plane B takes off from town P at the same time as A and flies to town R along the parallel of latitude.
(a) Calculate the shortest distance between towns P and Q in km.
(b) Find the distance in km traveled by plane B between P and R to the nearest km
(c) If the two planes fly at the same speed determine to the nearest minute, which plane Reaches its destination earlier and by how long.
(Take pi=22/7 and radius of earth R = 6370km)
Date posted: September 11, 2019. Answers (1)
- The figure below is a rectangular base pyramid with the base ABCD and vertex V.(Solved)
The figure below is a rectangular base pyramid with the base ABCD and vertex V.
VC=VB=VA=VD=39 AB=24 and BC = 18 EF = 8cm FG = 6cm
(a) Calculate the height VO of the Pyramid.
(b) The Pyramid is cut along a plane EFGH parallel to ABCD as shown.
(i) Calculate the angle between VHE and VBC
(ii) Calculate the angle between ABGH and ABCD
Date posted: September 11, 2019. Answers (1)
- A triangle T whose vertices are A (2,3) , B (5,3) and C (4,1) is mapped onto triangle T(Solved)
A triangle T whose vertices are A (2,3) , B (5,3) and C (4,1) is mapped onto triangle T whose vertices are A1 (-4,3), B1 (-1,3) and C1 (x, y) by transformation
(a) Find the
(i) Matrix M of transformation.
(ii) Find the coordinates of C
(b) Triangle T2 is the image of triangle T1 under a reflection in the line y = x. Find single matrix that maps T onto T2
(c) Find the coordinates of T2
Date posted: September 11, 2019. Answers (1)
- The quantities p and r are connected by the equation p = krn. The table of values of p and r is
Given below.(Solved)
The quantities p and r are connected by the equation p = krn. The table of values of p and r is
Given below.
(a) State a linear equation connecting p and r.
(b) Using the scale 2cm to represent 0.1 units on the y – axis and 1cm rep 0.1 units on the x – axis, draw a suitable line graph on the grid provided.
(c) Hence estimate the value of k and n
(d) Find the law connecting p and r
Date posted: September 11, 2019. Answers (1)
- (a) Complete the table below for the functions y = 2 Cos x and y = sin 2x (Solved)
(a) Complete the table below for the functions y = 2 Cos x and y = sin2x for -180º ≤ x ≤ 180º
(b) On the grid provided, draw on the same axis the graphs of y = 2cos x and y sin 2x for -180º ≤ x ≤ 180º
(c) Use the graphs in (b) above to find;
(i) The values of x such that 2 cos x - sin 2x = 0
(ii) State the amplitude and period of graph y = 2 cos x.
(iii) Find the difference in the value of y when x = - 450
Date posted: September 11, 2019. Answers (1)
- The taxation rate for 2007 were as in the table below.(Solved)
The taxation rate for 2007 were as in the table below.
In certain month in 2007, Ekachoro paid a tax of ksh.3000 after having been given a personal relief ksh.1056 per month.
(a) How much tax per month could he have paid if he did not get tax relief.
(b) Calculate his total taxable income in ksh. P.M.
(c) Given that his total taxable allowance amount to ksh.4520 p.m. Calculate his monthly basic salary in Kenya shillings.
Date posted: September 11, 2019. Answers (1)
- Given the co-ordinates of a point P is (-1, 3, 5) and Q is (2, -2, 4).(Solved)
Given the co-ordinates of a point P is (-1, 3, 5) and Q is (2, -2, 4). Find
(a) PQ in terms of i, j and k
(b) /PQ/
Date posted: September 11, 2019. Answers (1)
- Using a ruler and pair of compass only construct :
(a) An equilateral triangle ABC of side 6cm(Solved)
Using a ruler and pair of compass only construct :
(a) An equilateral triangle ABC of side 6cm
(b) The focus of a point P inside the triangle such that AP < or equal to PB
(c) The locus of a point Q such that AQ > 4cm
(d) Mark and label the region x inside the triangle which satisfy the two loci.
Date posted: September 11, 2019. Answers (1)