Get premium membership and access questions with answers, video lessons as well as revision papers.
Let x be the digit in the hundreds place and y be the digit in the units place, since the digit in the tens place is 0, then the total value of the number is:
100x + y
If the digits are reversed then total value of the number is:
100y + x
100y + x – (100 x + y) = 495
Difference then is: 99y – 99y = 495
y – x = 5 …………………….(i)
Sum of the other two digits
x + y = 11 ……………………….(ii)
From (i) and (ii) we find x = 3 and y = 8 hence the number is 308
Kavungya answered the question on September 24, 2019 at 12:21
- Use logarithm tables to evaluate(Solved)
Use logarithm tables to evaluate 
Date posted: September 24, 2019. Answers (1)
- Triangle ABC is such that A(-5, 1), B(-1, 1) and C(-3, 4). Triangle A'B'C'.is the image of ?ABC under transformation
a)Determine the co-ordinates of ?A'B'C'.
b)On...(Solved)
Triangle ABC is such that A(-5, 1), B(-1, 1) and C(-3, 4). Triangle A′B′C′.is the image of ∆ABC under transformation 
a)Determine the co-ordinates of ∆A′B′C′.
b)On the grid provided draw ∆ABC and ∆A′B′C′.
c)Describe the transformation T fully.
d)∆A′′B′′C′′ is a reflection of the ∆A′B′C′ on the line y = -x. Construct∆A′′B′′C′′.
e)Determine a single matrix that maps ∆A′′B′′C′′ onto ∆ABC.
Date posted: September 24, 2019. Answers (1)
- The figure shows a right pyramid mounted onto a rectangular block. The length AB=8cm, BC = 6cm, CH = 3cm and VC = 15cm
Given that...(Solved)
The figure shows a right pyramid mounted onto a rectangular block. The length AB=8cm, BC = 6cm, CH = 3cm and VC = 15cm
Given that M is the centre of the plane ABCD and P is a point on MV such that MP =1/5 MV, Calculate:-
(a)The length of EG.
(b)The height MV of the pyramidal section to 2 decimal places.
(c)The angle between the plane BPC and the pyramidal base ABCD.
(d)The angle between the line GV and the plane EFGH.
(e)The volume of the solid to four significant figures.
Date posted: September 24, 2019. Answers (1)
- Using a ruler and a pair of compass only;
a)Construct a triangle PQR such that PR=7.5cm, PQ=3.0cm and (Solved)
Using a ruler and a pair of compass only;
a)Construct a triangle PQR such that PR=7.5cm, PQ=3.0cm and b)Construct the locus T of points which are equidistant from a point L and passes through the vertices P, Q and R.
c)Locate the locus S on T such that it is equidistant from sides PQ and QR of the triangle.
d)Locate the locus of points G enclosed by PQ and QS such that QG <2cm
e)Measure SL.
Date posted: September 24, 2019. Answers (1)
- OPQ is a triangle in which OP=p and OQ=q. X is a point on OP such that OP: XP = 5: 2 and Y is...(Solved)
OPQ is a triangle in which OP=p and OQ=q. X is a point on OP such that OP: XP = 5: 2 and Y is another point on PQ such that PY: YQ = 1: 2. Lines OY and XQ intersect at T.
a) Express the following vectors in terms of p and q
(i)PQ
(ii)OY
(iii)QX
(b)If OT = k OY and QT = h QX express OT in two different ways. Hence or otherwise find the values of h and k.
(c) Determine the ratio OT: TY.
Date posted: September 24, 2019. Answers (1)
- (a) Complete the table given below by filling the blank spaces.
(b) On the grid provided draw on the same axes, the graph of y=4cos2x and...(Solved)
(a) Complete the table given below by filling the blank spaces.
(b) On the grid provided draw on the same axes, the graph of y=4cos2x and y=2sin(2x+300 for 00≤ x ≤ 1800
(c) From your graph
(i) State the amplitude of y=4cos2x
(ii) Find the period of y=2sin(2x+300
(d) Use your graph to solve 4cos2x-2sin(2x+300)0
Date posted: September 24, 2019. Answers (1)
- The table below shows the distribution of ages in years of 50 adults who attended a clinic
(a)State the median class
(b)Using a working mean of 45.5,...(Solved)
The table below shows the distribution of ages in years of 50 adults who attended a clinic
(a)State the median class
(b)Using a working mean of 45.5, calculate:-
(i)The mean age
(ii)The standard deviation
(iii)Calculate the 6thdecile.
Date posted: September 24, 2019. Answers (1)
- The average of the first and fourth terms of a GP is 185. Given that the first term is 27, find the common ratio.(Solved)
The average of the first and fourth terms of a GP is 185. Given that the first term is 27, find the common ratio.
Date posted: September 24, 2019. Answers (1)
- Solve for x in log(2x + 4) - log(x-1) = 3log 2.(Solved)
Solve for x in log(2x + 4) - log(x-1) = 3log 2.
Date posted: September 24, 2019. Answers (1)
- Make h the subject of the formula (Solved)
Make h the subject of the formula 
Date posted: September 24, 2019. Answers (1)
- Evaluate(Solved)
Evaluate 
Date posted: September 24, 2019. Answers (1)
- Solve the following linear inequalities and list the integral values of x.(Solved)
Solve the following linear inequalities and list the integral values of x.
Date posted: September 24, 2019. Answers (1)
- The equation of a circle is given by 4x2 + 4y2 +12x – 16y -11 = 0. Determine the radius and the co-ordinates of...(Solved)
The equation of a circle is given by 4x2 + 4y2 +12x – 16y -11 = 0. Determine the radius and the co-ordinates of the centre of the circle.
Date posted: September 24, 2019. Answers (1)
- The cost of maize flour and millet flour is Kshs 44 and Kshs 56 respectively. Calculate the ratio in which they were mixed if a...(Solved)
The cost of maize flour and millet flour is Kshs 44 and Kshs 56 respectively. Calculate the ratio in which they were mixed if a profit of 20% was made by selling the mixture at Kshs. 54.
Date posted: September 24, 2019. Answers (1)
- Solve for theta given that 2sin theta=tan theta for 00 less than or = theta less than or = 3600. (Solved)
Solve for theta given that 2sin theta=tan theta for 00 less than or = theta less than or = 3600.
Date posted: September 24, 2019. Answers (1)
- Given the expression 9.7 ÷ 3.7, find to four significant figures the percentage error in its quotient. (Solved)
Given the expression 9.7 ÷ 3.7, find to four significant figures the percentage error in its quotient.
Date posted: September 24, 2019. Answers (1)
- Evaluate using logarithms. (Solved)
Evaluate using logarithms.
Date posted: September 24, 2019. Answers (1)
- The data below shows length of leaves in a plant in mm. Complete the table.
Using D, calculate;
(i) The mean
(ii) The variance
(iii) The standard deviation(Solved)
The data below shows length of leaves in a plant in mm. Complete the table.
Using D, calculate;
(i) The mean
(ii) The variance
(iii) The standard deviation
Date posted: September 24, 2019. Answers (1)
- The relation between two variables P and V is believed to be of the form where A and B are constants.
(a)...(Solved)
The relation between two variables P and V is believed to be of the form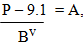 
where A and B are constants.
(a) Draw a suitable line graph and use it to determine the value of A and B
(b) Estimate the value of P when the value of V is 5.
Date posted: September 24, 2019. Answers (1)
- The manager of Hilton hotel has enough funds to buy at most 100 crates of soft drinks of type A and type B. He wishes...(Solved)
The manager of Hilton hotel has enough funds to buy at most 100 crates of soft drinks of type A and type B. He wishes to buy more than twice as many crates of type A as type B. He wants to buy a maximum of 80 crates of type A and at least 10 crates of type B. Letting x represent the number of crates of type A and y the number of crates of type B;
(a) Write down all the inequalities which represent the above information.
(b) Represent the inequalities in (a) above on the graph provided.
The profit from the sale of a crate of type A is kshs. 60, while that of type B is kshs. 40. Find the number of crates he should buy to maximize his profit.
(c) Find the profit.
Date posted: September 24, 2019. Answers (1)