- Make N the subject of the formula E =(Solved)
Make N the subject of the formula E =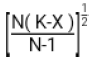 
Date posted: November 15, 2019. Answers (1)
- Simplify(Solved)
Simplify 
Date posted: November 15, 2019. Answers (1)
- A transformation M maps a rectangle OABC onto OAB’C’. Describe the transformation and write down the matrix for M.(Solved)
A transformation M maps a rectangle OABC onto OAB’C’. Describe the transformation and write down the matrix for M.
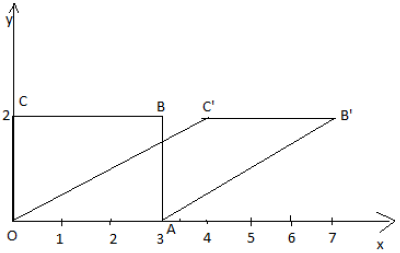
Date posted: November 15, 2019. Answers (1)
- Evaluate(Solved)
Evaluate 
Date posted: November 15, 2019. Answers (1)
- ABCD is a cyclic quadrilateral. Find k.(Solved)
ABCD is a cyclic quadrilateral. Find k.
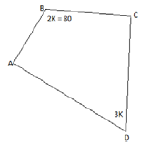
Date posted: November 15, 2019. Answers (1)
- Solve for x in 4 sinx – 4 cos2 x = -1 for 0 = x = 3600.(Solved)
Solve for x in 4 sinx – 4 cos2 x = -1 for 0 ≤ x ≤ 3600.
Date posted: November 15, 2019. Answers (1)
- P is ( 12,-6, 9) and Q (3, 18, -12). A is the point which divides PQ in the ratio 2: 1. Find the coordinates...(Solved)
P is ( 12,-6, 9) and Q (3, 18, -12). A is the point which divides PQ in the ratio 2: 1. Find the coordinates of A and express PA in terms of i ,j and k.
Date posted: November 15, 2019. Answers (1)
- The matrix maps a quadrilateral with area 5 cm2 on to a quadrilateral with area 25 cm. Find the possible value 25 cm2. Find...(Solved)
The matrix 
maps a quadrilateral with area 5 cm2 on to a quadrilateral with area 25 cm. Find the possible value 25 cm2. Find the possible values of x.
Date posted: November 15, 2019. Answers (1)
- Solve the equation log 3 + log (4x + 4) = 1 + log (2x – 2).(Solved)
Solve the equation log 3 + log (4x + 4) = 1 + log (2x – 2).
Date posted: November 15, 2019. Answers (1)
- A curve whose gradient function is dy/dx = 3x2 +10x +3 passes through point (-2, -3)
a) Find the equation of the curve
b) i) Determine...(Solved)
A curve whose gradient function is dy/dx = 3x2 +10x +3 passes through point (-2, -3)
a) Find the equation of the curve
b) i) Determine the turning points
ii) Sketch the curve
Date posted: November 14, 2019. Answers (1)
- a) Find M-1 , the inverse of Mb) In the first term of a certain year, a mixed school bought sixty pairs of girls’...(Solved)
a) Find M-1 , the inverse of M 
b) In the first term of a certain year, a mixed school bought sixty pairs of girls’ socks and ninety pairs of boys’ socks for a total of Ksh 14,100. In the second term of the same year the school bought.
30 pairs of girls’ socks and 50 pairs of boys’ socks for a total of Ksh 7600.
(i) Form a matrix equation that represent the information above
(ii) Use the matrix M-1 to find the price of a pair of each type of socks
c) In the third term of the year, the supplier gave a discount of 10% on each of pair girls’ socks and a discount of 20% on each pair of boys’ socks. If the school bought 25 pairs of girls’ socks and 40 pairs of boys’ socks in the term, find the percentage discount on the cost of all the socks that were bought.
Date posted: November 14, 2019. Answers (1)
- The table below shows the number of letters collected from the post office by a school messenger during a school year.
(i) Estimate the median of...(Solved)
The table below shows the number of letters collected from the post office by a school messenger during a school year.
(i) Estimate the median of this data.
(ii) Estimate the mean of this data.
(iii) On the grid provided, draw a histogram and a frequency polygon to represent this data.
Date posted: November 14, 2019. Answers (1)
- The diagram below represents a conical vessel which stands vertically. The vessels contains water to a depth of 30cm. The radius of...(Solved)
The diagram below represents a conical vessel which stands vertically. The vessels contains water to a depth of 30cm. The radius of the surface in the vessel is 21cm. (Take ?? = 22/7)
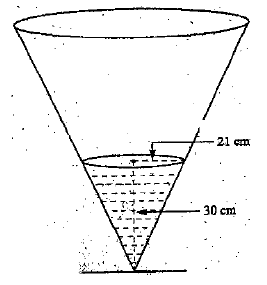
a) Calculate the volume of the water in the vessels in cm3
b) When a metal sphere is completely submerged in the water, the level of the water in the vessels rises by 6cm.
Calculate:
i) The radius of the new water surface in the vessel;
ii) The volume of the metal sphere in cm3
ii) The radius of the sphere
Date posted: November 14, 2019. Answers (1)
- (a) Draw the graph of y = 2 + 3x - x2 in the range of - 3 = x = 6 on the grid...(Solved)
(a) Draw the graph of y = 2 + 3x − x2 in the range of − 3 ≤ x ≤ 6 on the grid provided.
b) From your graph:-
(i) Find the value of x if x2 − 4x = 0
(ii) Determine the value of x for which y is the greatest.
(iii) Determine the range of values of x for which y is positive.
Date posted: November 14, 2019. Answers (1)
- Draw a triangle ABC such that angle BAC = 90°, AC =5 cm and BC = 10 cm.
i. Circumscribe a circle on the triangle...(Solved)
Draw a triangle ABC such that angle BAC = 90°, AC =5 cm and BC = 10 cm.
i. Circumscribe a circle on the triangle
ii. Measure the radius of the circle.
iii. Find the difference in the area of the circumcircle and the triangle.
Date posted: November 14, 2019. Answers (1)
- The diagram below represents the cross- section of a solid prism of length 3m.
Calculate the volume of the prism in cm3.(Solved)
The diagram below represents the cross- section of a solid prism of length 3m.
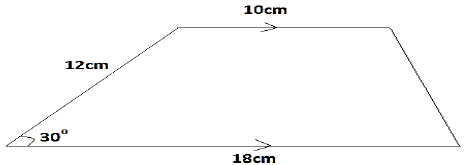
Calculate the volume of the prism in cm3.
Date posted: November 14, 2019. Answers (1)
- Simplify the expression given by(Solved)
Simplify the expression given by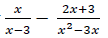 
Date posted: November 14, 2019. Answers (1)
- Use square, square root and reciprocal tables only to evaluate the following giving your answer to 2 decimal places.(Solved)
Use square, square root and reciprocal tables only to evaluate the following giving your answer to 2 decimal places.
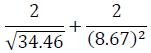
Date posted: November 14, 2019. Answers (1)
- Given that sin theta = 3/5 and 900 = < = 1800 , find without using trigonometric tables, the value of 2 tan theta + 3 cos theta.(Solved)
Given that sin theta = 3/5 and 900 = < = 1800 , find without using trigonometric tables, the value of
2 tan theta + 3 cos theta.
Date posted: November 14, 2019. Answers (1)
- Form three inequalities that satisfy the unshaded region R.(Solved)
Form three inequalities that satisfy the unshaded region R.
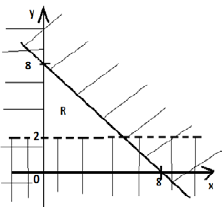
Date posted: November 14, 2019. Answers (1)